Mathematics, often regarded as a formidable subject, can be an exhilarating journey of exploration and discovery. At its core lies the art of problem-solving, a skill that transcends equations and numbers. Developing problem-solving skills in math is not only essential for excelling academically but also for nurturing critical thinking, logical reasoning, and creativity.
It equips individuals with the ability to tackle real-life challenges and make informed decisions. In this blog post, we will delve into effective strategies for solving challenging math problems, empowering you to overcome obstacles and embrace the beauty of mathematics.
Understand the problem: Analyzing the given information and requirements
Before embarking on the journey of problem-solving, it is crucial to comprehend the problem at hand. Read the situation carefully, identifying key information and discerning the requirements. Consider what is known and what is unknown, and determine the relationships between the given elements.
Take note of any constraints or conditions that might influence the solution. By breaking down the trouble into its fundamental components, you can gain a clear understanding of what needs to be solved. Remember, while seeking assistance online can be beneficial for learning, it is important to approach it responsibly.
Websites like “deltamath answers” can provide additional resources and explanations, but it is essential to use them as tools to enhance your understanding and not as a substitute for the problem-solving process.
Identify relevant concepts: Recognize the mathematical principles applicable to the problem
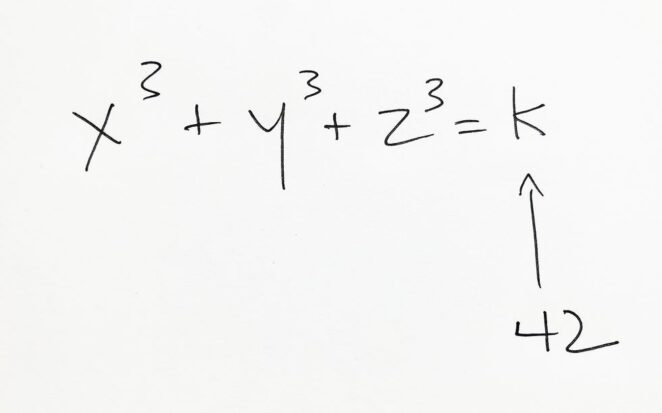
Mathematics is built upon a vast web of interconnected concepts and principles. Once you understand the concern, it is time to identify the relevant mathematical concepts that can be applied to solve it. Draw upon your knowledge of arithmetic, algebra, geometry, or calculus, depending on the nature of the trouble.
Recognize patterns and relationships that align with the given issue, and leverage appropriate formulas or theorems. This step is crucial in bridging the gap between the problem statement and the tools available to solve it.
Break it down: Breaking the problem into smaller, manageable parts
Complex math problems can often appear overwhelming at first glance. To avoid feeling inundated, break down the issue into smaller, more manageable parts. Analyze the crisis step by step, unraveling its layers of complexity.
Divide the situation into subproblems or stages, each with its own set of objectives. By tackling one piece at a time, you not only make the concern less daunting but also create a clear roadmap toward the solution.
Use visual aids: Diagrams, graphs, and charts to visualize the problem
Visual aids are powerful tools in solving, enabling you to visualize and comprehend the issue more effectively. Employ diagrams, graphs, charts, or any other visual representation that aligns with the issue context.
Visualizing the information can provide valuable insights and reveal hidden patterns or relationships. A geometric situation, for instance, can benefit greatly from a carefully constructed diagram. By leveraging visual aids, you enhance your understanding of the issue and pave the way for innovative solutions.
Explore different strategies: Try multiple approaches to find the most effective solution

A single problem can often be approached in various ways. Exploring different strategies allows you to expand your problem-solving repertoire and discover the most effective solution. Start by applying conventional methods that align with the requirements.
Then, venture into uncharted territories, experimenting with alternative approaches or creative techniques. Embrace a growth mindset, as each strategy attempted, successful or not, adds depth to your mathematical toolbox and cultivates adaptability.
Make connections: Relating the problem to previous knowledge and similar examples
Mathematics is an interconnected tapestry, where each concept builds upon those that came before. When facing a challenging issue, tap into your previous knowledge and experiences. Seek connections between the current situation and similar examples you have encountered in the past.
Analogies, patterns, or even personal experiences can offer valuable insights and alternative perspectives. By making connections, you leverage your existing knowledge base, enriching your difficulty-solving process with a broader range of approaches.
Trial and error: Experimenting with different approaches and learning from mistakes
Mathematics, like any other discipline, embraces trial and error as an inherent part of the learning process. Do not shy away from experimenting with different approaches, even if they might lead to incorrect solutions initially. Mistakes are opportunities for growth and learning.
Embrace them as stepping stones on your path to success. Analyze the errors you encounter, identify their causes, and learn from them. By refining your strategies through continuous experimentation, you sharpen your problem-solving skills and develop a deeper understanding of mathematical concepts.
Seek help and collaborate: Consulting teachers or peers for guidance and fresh perspectives

Problem-solving is not a solitary endeavor. Seeking help and collaborating with others can provide fresh perspectives and insights that you might have missed. Engage with your teachers, who possess a wealth of knowledge and expertise.
Consult peers who share your passion for math, as they can offer unique approaches and support. Through collaboration, you foster a stimulating environment that encourages innovative thinking and nurtures a growth mindset. Embrace the opportunity to learn from others and collectively conquer challenging math issues.
Practice regularly: Engaging in regular problem-solving exercises to build proficiency
Like any skill, problem-solving in math requires practice to build proficiency. Engage in regular situation-solving exercises, challenging yourself with a diverse range of concerns. Solve puzzles, work through textbooks, or explore online resources that offer difficulty sets.
Embrace the journey, rather than focusing solely on the destination. Celebrate small victories and learn from each issue you encounter. With consistent practice, you develop a deep intuition for difficulties-solving, honing your skills, and fortifying your mathematical foundation.
Reflect and learn: Evaluating the solution process and applying insights to future problems

Reflection is the key to growth and improvement. Once you have solved an issue, take the time to evaluate your solution process. Assess the effectiveness of the strategies employed, the clarity of your explanations, and the accuracy of your calculations.
Identify areas for improvement and consider how you can apply the insights gained to future issues. Embrace a growth mindset that embraces continuous learning and adaptation. Each issue you solve becomes a stepping stone towards becoming a more proficient and confident concern solver.
Conclusion
In conclusion, developing problem-solving skills in math is an empowering endeavor that opens the doors to a world of possibilities. By following these strategies—understanding the problem, identifying relevant concepts, breaking it down, using visual aids, exploring different approaches, making connections, engaging in trial and error, seeking help and collaboration, practicing regularly, and reflecting on the process—you can conquer challenging math situations with confidence. Embrace the journey of hardship-solving, and let the beauty and elegance of mathematics unfold before your eyes.




